In economics, the formula for average variable costs gives the insight of a person as to how businesses will determine their production efficiency regarding variable expenses. The average variable cost, in other words, is the variable per-unit production cost. It can be calculated through total variable cost divided by the quantity produced, Q. That is to say, this formula will allow a business to analyze the profit it makes whenever production increases or decreases. Thus, it is crucial for decision-making, pricing, and output levels.
What is Average Variable Costs Formula?
The average variable cost is calculated as the total variable costs to units produced. Mathematically, it can be written as:
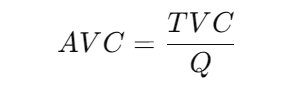
Where:
- AVC = Average Variable Cost
- TVC = Total Variable Costs
- Q = Quantity of goods produced
Variable costs are those costs that vary with the changes in the level of operation. Examples include raw materials, labor, and utilities used to produce an item. Average variable cost is very important in establishing the behavior of variable costs concerning increases or decreases in output and, at the same time, optimizing the extent of production efficiency.
Importance of Average Variable Costs Formula
- Decision Making: AVC is utilized to determine whether or not the production cycle should continue or be halted. Depending on which is more convenient to stop production, if AVC exceeds the selling price of the product, then it will be preferred to halt production.
- Break-even Analysis: It helps in determining the point at which the revenue generated is equal to the variable costs of production.
- Cost Management: AVC trend analysis will inform the companies where they can cut their variable costs.
Average Variable Cost Formulas
Different methods express the average variable cost formula, which depends on the context of a business while doing its cost analysis. The most common forms include:
Basic Formula:
AVC = TVC / Q
This is the fundamental formula where AVC is simply the total variable cost divided by the output quantity.
Using Marginal Cost:
Marginal cost (MC) is the cost of one extra unit of product. Now, use AVC to predict future AVC. Such a forecasting of AVC can be made when AVC = MC. This implies that the company is in an efficient operation.
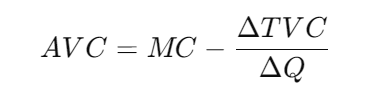
This variation accounts for changes in variable costs with respect to production levels.
Average Variable Cost with Fixed Costs:
In cases where both fixed and variable costs are analyzed, the total cost (TC) is broken down:
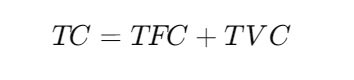
Here, the total cost is the sum of total fixed costs (TFC) and total variable costs (TVC). The AVC is derived from the TVC part.
Methods to Calculate Average Variable Cost
There are different approaches to calculating AVC, depending on the availability of data and the specific objectives of the analysis.
1. Direct Calculation from Total Variable Costs
The easiest method is to directly use the formula:
AVC = TVC / Q
This method works when total variable costs are readily available. For instance, if the total variable cost to produce 100 units is $1,000, the AVC is:
AVC = 1,000 / 100= 10 per unit
2. Marginal Cost Approach
Another way is to derive AVC from marginal costs. Marginal cost measures the increase in total cost resulting from the production of an extra unit. Thus, such a method is appropriate when incremental production costs are analyzed.
3. Break-even Analysis
Since AVC is introduced in the break-even analysis, it can be noted how many units would have to be produced and sold to recover even both fixed and variable costs. Its break-even formula is as follows:
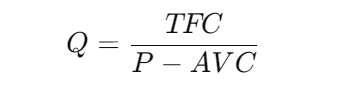
Where:
- Q = Break-even quantity
- TFC = Total fixed costs
- P = Price per unit
- AVC = Average variable cost

Tips for Calculating Average Variable Cost
AVC must then be calculated for proper use in financial planning, pricing strategy, and general cost controls. For simplicity, the following are tips about finding AVC:
- All variable costs correctly identified: Before the calculation of AVC, all variable costs should be accurately described. These costs must vary with the level of production.
- Fixed costs excluded: Fixed costs form no part of AVC. Examples include rent, permanent salaries, and depreciation, which are considered not to vary with the levels of production.
- Use the Marginal Cost for Insight: For variable costs that happen in unpredictable ways, one can compare AVC with marginal cost and understand better the pattern of cost behavior at different levels of production.
- Consider Economies of Scale: For economies of scale in big-batch production, AVC may be reduced even further. Consider if economies of scale can be exploited by increasing the batch size to obtain lower AVCs.
- Track Trends Over Time: AVC provides a means for monitoring trends over time so that future cost trends can be predicted and the necessary changes made in terms of production and pricing.
Conclusion
Understanding how to apply the average variable costs formula is basically at the heart of business cost management. Knowing one’s way around a function for calculating AVC can determine profitability, ensure the right production decisions, and control variable costs. Regardless of whether one uses the basic formula or other more complex ways of finding it, AVC provides information crucial to knowing how one has structured the costs of a company.
Average Variable Costs Formula FAQs
What are average variable costs?
Average variable costs refer to the per-unit variable cost of production, calculated by dividing total variable costs by the quantity of goods produced.
How do fixed and variable costs differ?
Fixed costs do not change with the level of production, while variable costs fluctuate directly with production volume.
Can average variable costs decrease over time?
Yes, AVC can decrease due to economies of scale, where higher production volumes lead to lower per-unit costs.
Why is the average variable cost formula important?
The formula is crucial for analyzing cost efficiency, determining break-even points, and making decisions on production levels.
How is the average variable cost different from total cost?
While AVC focuses solely on variable costs, total cost includes both fixed and variable expenses.


